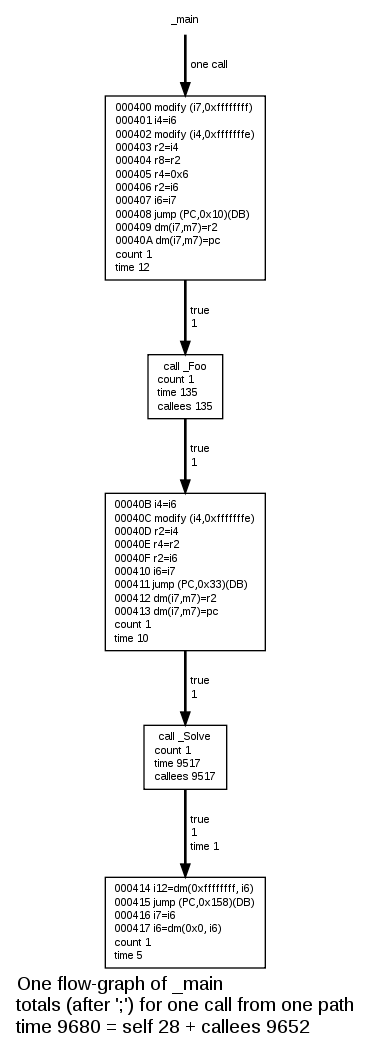
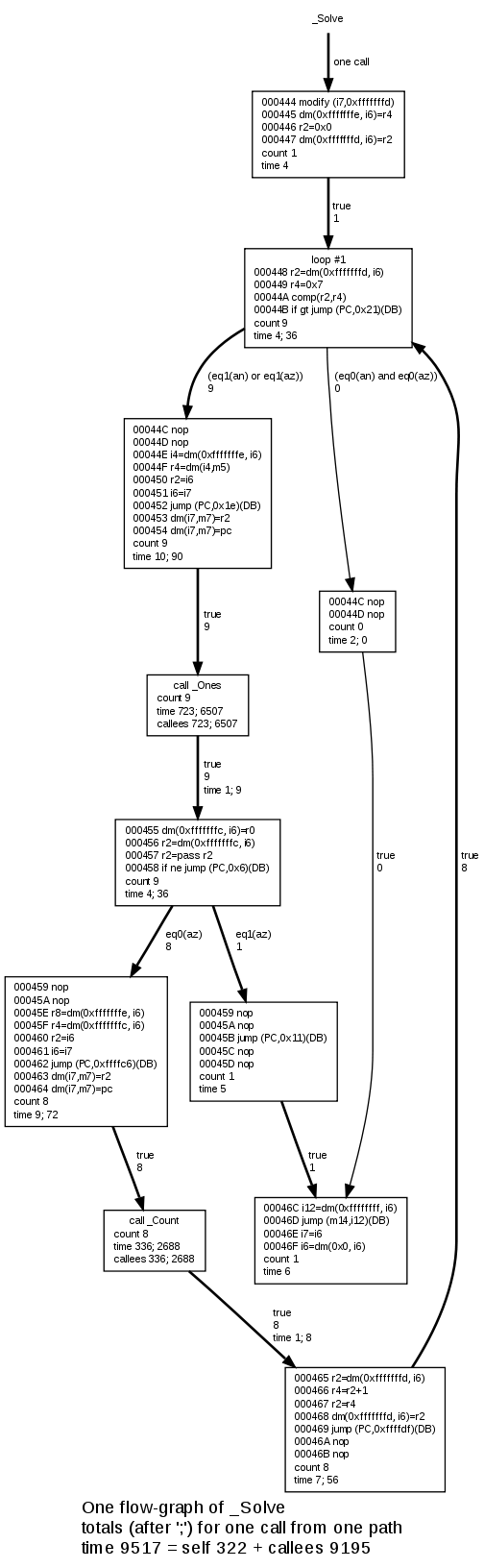
Bound-T can draw control-flow graphs (or flow graphs, for short) of the analysed subprograms. The image on the right shows the simple flow graph of the main subprogram. It begins with the subprogram name at the top and an arrow from the name, to the box that represents the entry point of the subprogram.
Most boxes in a flow-graph diagram represents basic blocks of code. A basic block is a sequence of instructions that is entered only at the first instruction and left only at the last instruction. Depending on command-line options Bound-T can label (fill in) the box with various data about the basic block. In this example we have used the option "-draw decode" to include the disassembled instructions and their addresses, and the option "-draw no_line" to omit the name of the source-code file and the numbers of the source lines that give rise to these instructions. The last two text lines in each box show the number of times this basic block is executed in the worst-case scenario ("count") and the WCET bound ("time").
The other boxes in a flow-graph diagram represent the execution of other subprograms due to calls from the present subprogram. These boxes are labelled with "call <callee name>" followed by the execution "count" and the execution "time" bound. The last line in the box for a callee shows the part of the time that is spent in the callee (which currently is all of the "time" for this box; in a future version of Bound-T there will be an option to compress the flow-graphs by combining these call boxes with the basic-block box that contains the call instruction, and then this "callees" label will be significant).
The arrows show the flow of control between the basic blocks and callees. The labels on the arrows show the logical condition of taking this arrow and how many times the arrow is executed in the worst-case scenario. Since the main subprogram has no conditional branches all the conditions are "true", and all blocks and arrows in its flow graph are executed once.
A box that has no arrows leaving it represents a basic block that ends by returning from the subprogram. In the main function the bottom block in the graph at right is a return block.
One context, or summary total of all contexts
A flow-graph drawing can represent one execution context of the subprogram, or the total of all execution contexts of this subprogram. To be precise, in the first case the flow graph shows the subprogram's execution bounds for one context, while in the second case it shows the sum total of all calls to this subprogram, in all contexts, that are included in the worst-case scenario. The flow graph of main shows one execution context of main (and this is the only execution context since main is the root subprogram of this example analysis).
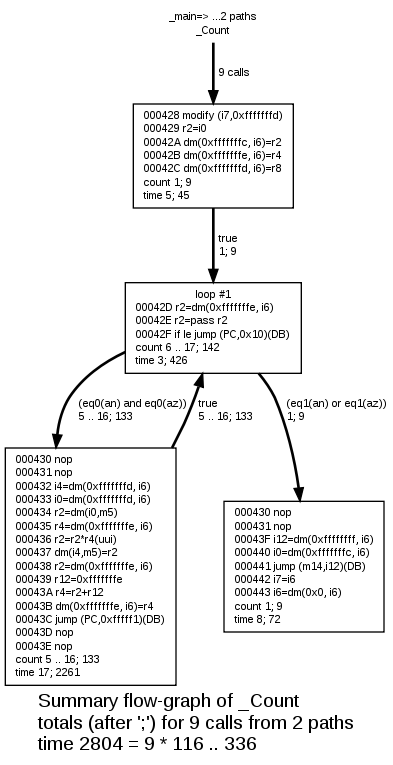
A "total" flow-graph drawing differs from a one-context drawing only when the subprogram has context-specific execution bounds in the analysis. In this example program this happens only for the subprogram Count, which has different execution bounds when it is called from subprogram Foo (when Count takes at most 116 cycles) and when it is called from subprogram Solve (when Count takes at most 336 cycles). This is illustrated in the context-specific form of the call-graph drawing.